Originally Posted by Brian Rush

All you're doing is presenting a moral argument that the skimmer deserves to do the skimming. The fact that he's skimming from the labor of others remains a fact, regardless of how you feel about that personally. Profit is in a different existential category from pay for work, no matter how much the work is paid, and so the comparison between someone who works with hands and muscles and someone who pushes paper around a desk is not pertinent.

I could in fact argue with your moral position. Bill Gates didn't make money from Microsoft because he knew what needed to be done and instructed the employees to do it. He made money from Microsoft because he owned the business. If he had sat back and let someone else do the job that he did as CEO, he'd have still owned Microsoft and still made money from it. (Come to think of it, now that he's retired, that's exactly what he does.) If the hired CEO had done a better job, he might even have made more money for being a lazy slob than he did for working at the business.

And by the way -- once again, it has nothing to do with me. "More successful than me" is one hundred percent irrelevant. If you were right, I would not be making a distinction between those who make their money in profit, and those who are paid high salaries for their work, such as sports stars or movie stars, who also make more money than I do. I've seen before that conservatives seem to have a problem understanding anyone who cares about society in general, since they don't. Perhaps everything is personal and selfish for you. It's not for me. And it's also telling that when you don't have a good answer for something, you always seem to fall back on personal insults, which of course this is -- unwarranted, untrue, and frankly contemptible. You should be ashamed.

Alrighty then. Sometimes I forget how extreme the people I'm talking to here are, and then BAM I'm reminded. If you want to get rid of "profit", what you're saying is that all income and resulting wealth belongs to the government, and the government should decide how it's used. Period. No private investment. All directed by the government. There are communist countries that don't even go that far.

Originally Posted by btl2283

Eliminating the Bush tax cuts for everyone would actually close 75 percent of the budget deficit over the next five years.

For everyone, in all brackets, possibly. That is, if it didn't send the economy into a steep recession, negating the revenue it would theoretically have brought in. Which it almost certainly would. I encourage the Democratic Party to pursue that policy as part of its agenda.

Originally Posted by JustPassingThrough

If you want to get rid of "profit"

Don't jump the gun, please. I haven't suggested any specific reforms in this discussion. All I'm doing at the moment is resisting the lionization of people who don't deserve it, and pointing out that a great deal of the money that people acquire and own in our economy is actually earned, in any reasonable sense of that word, by others on their behalf.

And you're engaging in a sole-alternative fallacy. There are plenty of possibilities other than kow-towing to the owner class and extreme state socialism of the sort you described. What's more, you know that.

Originally Posted by JustPassingThrough

For everyone, in all brackets, possibly. That is, if it didn't send the economy into a steep recession, negating the revenue it would theoretically have brought in. Which it almost certainly would. I encourage the Democratic Party to pursue that policy as part of its agenda.

How very "Keynsian" of you. I agree that right now stimulating a depressed economy takes priority over the deficit, which is more of a long term issue. That being said, the economy did fine under the Clinton era tax rates in the 90's, and the European economies do fine under higher levels of taxation, so there is no reason why it is not a longer term solution for a long term problem.

Originally Posted by Brian Rush

Don't jump the gun, please. I haven't suggested any specific reforms in this discussion. All I'm doing at the moment is resisting the lionization of people who don't deserve it, and pointing out that a great deal of the money that people acquire and own in our economy is actually earned, in any reasonable sense of that word, by others on their behalf.

And you're engaging in a sole-alternative fallacy. There are plenty of possibilities other than kow-towing to the owner class and extreme state socialism of the sort you described. What's more, you know that.

Under your moral framework, if a farmer who owns a tractor that he's only using 5 days a week sells his services to someone else on the other two days, takes his tractor over to their place and drives it himself, that's OK. But if he rents that tractor out and takes the weekend off while the person he rents it to does the driving, what he's doing is evil.

You can only achieve an economy consistent with your view of morality if you have complete government ownership of the means of production, and ultimately no private property. If that's what you believe, I'm not going to argue with you. There's no point.

My I suggest a few things:

1. Taxes are paid by people who are at work. Such people are actually doing quite well at the moment, or were until the gas explosion.

2. Taxes during a recession/depression take people away from money who have it--especially high high-income marginal tax rates--and can be paid to people who don't in exchange for public works jobs, helping recovery.

3. At some point, people are once again going to realize that creating billionaire fortunes serves no useful purpose.

Originally Posted by btl2283

How very "Keynsian" of you. I agree that right now stimulating a depressed economy takes priority over the deficit, which is more of a long term issue. That being said, the economy did fine under the Clinton era tax rates in the 90's, and the European economies do fine under higher levels of taxation, so there is no reason why it is not a longer term solution for a long term problem.

Like I said, I encourage the Democratic Party to run on the platform of tax increases for everyone. I think it will go well for them.

On the topic of the thread, I'm watching Sarah Palin on Sean Hannity's show right now. With the recent events in the Republican field -- Trump and Huckabee dropping out, Gingrich putting his foot in his mouth right out of the gate -- I think the chances of her running are increasing dramatically. She's been laying low, and I think a lot of people have written her off, but I have a feeling that assumption is premature.

Originally Posted by JustPassingThrough

On the topic of the thread, I'm watching Sarah Palin on Sean Hannity's show right now. With the recent events in the Republican field -- Trump and Huckabee dropping out, Gingrich putting his foot in his mouth right out of the gate -- I think the chances of her running are increasing dramatically. She's been laying low, and I think a lot of people have written her off, but I have a feeling that assumption is premature.

Say what you want about her, she knows how to work the media, her fans and has good dramatic timing for the camera, so I say you are right.

Originally Posted by btl2283

How very "Keynsian" of you. I agree that right now stimulating a depressed economy takes priority over the deficit, which is more of a long term issue. That being said, the economy did fine under the Clinton era tax rates in the 90's, and the European economies do fine under higher levels of taxation, so there is no reason why it is not a longer term solution for a long term problem.

I don't see killing the Bush tax cuts as being unwise in the least. Tax policy that creates little incentive to spend is of little benefit, and these cuts certainly qualify. If they were of any stimulative use, they would have created an overheated economy, rather than one that has under performed from 2001 to the present.

We have ~ $8 Trillion worth of spending that is needed ... some of it desperately. Let's get on with that. Spending is a much better stimulus, and the real #1 issue is not excessive debt or the bond vigilantes, it's jobs!

Originally Posted by JustPassingThrough

Like I said, I encourage the Democratic Party to run on the platform of tax increases for everyone. I think it will go well for them.

It actually might this time. Nothing is sacred forever. We've seen how the other model works, and it's pretty poor as a wealth creator ... except for the very few.

Originally Posted by JustPassingThrough

On the topic of the thread, I'm watching Sarah Palin on Sean Hannity's show right now. With the recent events in the Republican field -- Trump and Huckabee dropping out, Gingrich putting his foot in his mouth right out of the gate -- I think the chances of her running are increasing dramatically. She's been laying low, and I think a lot of people have written her off, but I have a feeling that assumption is premature.

I'll bet you $100 that she doesn't make a serious run if she runs at all. She's strictly in it for the money.

Sure enough:

Mitt Romney, Sarah Palin jump to top in new Gallup poll
Is Sarah Palin The Real GOP Frontrunner?
Palin Fundraiser Fuels Speculation of 2012 Run


I think recent events have begun to make the Republican field a lot more clear, and Palin is increasingly the only who can step in and solidify the conservative base and Tea Party elements, while also having the name recognition and fundraising ability to mount a strong campaign. I suspect it could come down to Palin vs. Romney. And if that's the way it ends up, Romney wouldn't stand a chance.

Originally Posted by Marx & Lennon

I'll bet you $100 that she doesn't make a serious run if she runs at all. She's strictly in it for the money.

I disagree. I think she's afraid to run because of the way the way the left and the media have relentlessly savaged her and her family for the last 2+ years. But now, when you see that the same thing is happening to every other Republican candidate, and they're imploding under the pressure, the fact that she's already been through the ringer and there's not much more they can do to her actually makes her stronger than the other options.

Originally Posted by Marx & Lennon

It actually might this time. Nothing is sacred forever. We've seen how the other model works, and it's pretty poor as a wealth creator ... except for the very few.

Ask Walter Mondale.

Originally Posted by Marx & Lennon

It actually might this time. Nothing is sacred forever. We've seen how the other model works, and it's pretty poor as a wealth creator ... except for the very few.

This is really the point. We've tried the Republican model for thirty years, created gigantic wealth inequality, seen the outsourcing of millions of jobs, and seen the incomes of the lower half of the population stagnate at best. The reason people like JPT are on my ignore list is that they won't admit the obvious: this is the result they want. Why I am not sure, since I don't think he's a multibillionaire himself--but it makes him feel good. I don't need to argue with people like that.

Originally Posted by JustPassingThrough

On the topic of the thread, I'm watching Sarah Palin on Sean Hannity's show right now. With the recent events in the Republican field -- Trump and Huckabee dropping out, Gingrich putting his foot in his mouth right out of the gate -- I think the chances of her running are increasing dramatically.

Wishful thinking -- with that and $2.12 you can get a $2 cup of coffee in Michigan (6% sales tax added). Without the wishful thinking you get the same cup of coffee. Without the $2.12 you don't get the cup of coffee with your misguided optimism.

She's been laying low, and I think a lot of people have written her off, but I have a feeling that assumption is premature.

That's lying low unless you are referring to sex. Sure, the gerund lying has suggestions of deliberate or reckless deceit, but she is a pathological liar anyway.

...She has castigated our President as a "professor of Constitutional Law instead of a leader", but for good reason we have elected lots of attorneys as President, and not other smart people like physicians, research scientists, engineers, novelists, journalists, and CPAs. Attorneys are generalists who show attention to critical details that make the difference between winning and losing a case. The only other group clearly over-represented in the Presidency are Army generals who are much the same except for the 'case' being the winning or losing of critical battles (Yorktown, Vicksburg, Normandy).

A sloppy thinker like Dubya, who would have never cut it as an attorney, would never have pulled off the assassination of Osama bin Laden -- and neither would she. I am glad that we now have a President who contemplates the consequences of his deeds and knows when to pay attention to details and when to delegate them, and insists upon a high quality of planning and execution by subordinates.

Originally Posted by JustPassingThrough

For everyone, in all brackets, possibly. That is, if it didn't send the economy into a steep recession, negating the revenue it would theoretically have brought in. Which it almost certainly would. I encourage the Democratic Party to pursue that policy as part of its agenda.

But could't making steep spending cuts also throw the economy back into recession?

Originally Posted by pbrower2a

Wishful thinking -- with that and $2.12 you can get a $2 cup of coffee in Michigan (6% sales tax added). Without the wishful thinking you get the same cup of coffee. Without the $2.12 you don't get the cup of coffee with your misguided optimism.

My assessment is neither optimism nor pessimism. I have my doubts about her, but it is increasingly clear that the other candidates are even more flawed. You may think it's impossible that she could win the general election, but I'm just talking about the Republican primary.

Originally Posted by JustPassingThrough

The really interesting fact about tax rates and revenue is that the amount of revenue going into government has been remarkably stable over time, regardless of the tax rates. Which is about as much proof of the basic concept behind the Laffer Curve as you could ask for.



Clearly, tax rates do not remotely have a direct relationship to the amount of revenue the government takes in.

This graph is for numbskulls.

The numerator is tax revenue and the denominator is GDP.

Economy is doing great (or, terrible), and, in synch, tax revenues go up (or, down) - numerator goes up(or, down) and, in synch, the denominator goes up (or, down), what value do you get (either way)? Constant. Duh.

The only significant shift in that period is in the mid-1940s. Gee, what happen then? Do you think it might be a tad more expensive to have become the world's superpower after WW2? Duh.

From this chart, one could say tax rates don't matter, but one could also then say economic recessions and booms don't matter, wars don't matter, politics don't matter, policy doesn't matter, people don't matter - you name it, doesn't matter.

Like I said, a chart for numbskulls.

- and for the numbskulls that think they know something about economics, you might want to look up the meaing of this word - "endogeneity"

An update on the sources of the federal deficit -



And for those with good memories, remember when CBO in 2000 projected an increasing surplus and there was concern for what negative consequences that would have? Well then came the pinnacle of movement conservatism, with its Laffer Curve, Supply Side, Ayn Rand, Social Darwinism idiocy in the form of George Bush Jr, who sure 'saved us' from those consequences -

Originally Posted by btl2283

Eliminating the Bush tax cuts for everyone would actually close 75 percent of the budget deficit over the next five years. Winding down our military committments in Afghanistan and Iraq, as we are planning to do, would eliminate the rest.

These may well be the right thing to do (though I don't think the Bush tax cuts should be repealed for the middle and lower classes), but even so, do these "estimates" include the hidden costs of these things? Does it include at least a modest decrease in taxable activity? Does the winding down of military operations and defense in general consider the impact on regions and metro areas heavily dependent on the presence of military bases and large defense contractor sites? Some areas were devastated by the Clinton-era base closures, for example, and a full, honest assessment of "cost savings" from these closures needs to be offset by the loss of tax revenue from individuals and businesses hard hit by the closure, not to mention the additional public assistance costs of helping those made unemployed or underemployed by the cuts.

Again -- not saying some of this is a terrible idea for that reason, just that numbers like these that tout "savings" need to consider the hidden cost of unintended consequences to offset the savings. It's like people who want to tax the hell out of "unhealthy" lifestyles because of the added cost they put on public health care, but these people usually fail to offset that by savings to Social Security and public pensions because these folks die years sooner on average.

Here's a very interesting chart comparing growth in GDP during periods of different top tax margins -


From AngryBear here -

http://www.angrybearblog.com/2010/12...ates-real.html

The chart below shows tax rates on one axis and the growth rates in real GDP that accompanied those tax rates on the other:



I broke the tax ranges into 5 percentage point increments centered around intuitive numbers (30%, 35%, etc.). Growth rates are the median observed for each range. For ranges which did not occur in the real world, growth rates are left blank.

Top marginal tax rates come from the IRS' Statistics of Income Historical Table 23, and are available going back to 1913. Real GDP can be obtained from the BEA's National Income and Product Accounts Table 1.1.6, and dates back to 1929. Thus, the graph uses data starting in 1929.

Consider this post a quick follow up to my previous post on optimal tax rates that appeared at the Presimetrics blog and Angry Bear blogs. There will be a lot more follow-ups, but it occurs to me that a look a the data might be useful before going on.

I bolded that last line for you, JPT, as perhaps some useful advice in life.

It's a cute picture, pw. But it makes very strong implications about causality's arrow that are almost certainly unfounded. Hell, absent at least an attempt at some sort of variance analysis, it's just shapes on a screen.

More lies via graphs is all any reasonably rational person would conclude from that above alone.

For those who will now wipe out their Barro to counter, don't bother. I got it covered -

http://www.angrybearblog.com/2011/05...enerating.html

Optimal Tax Rates for Generating Economic Growth According to Barro-Sahasakul Tax Data

This piece is a bit more wonky than what I normally post.

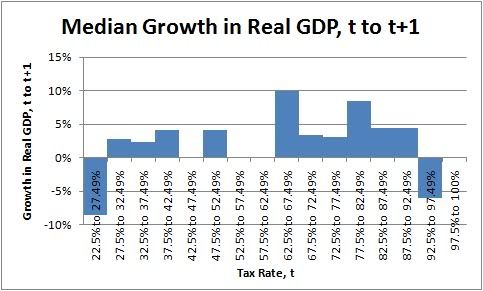
I recently re-read "Macroeconomic Effects from Government Purchases and Taxes" by Barro & Redlick. I was struck by how different the conclusions they make about taxes are from what you get if you simply make a bar chart of the top marginal rate at any given time versus the growth rate over the next year.

Now, obviously, Barro & Redlick take a completely different approach... but at the bottom of everything is the data set they use (see Table 1 of the above referenced paper and this explanation of the "Barro-Sahasakul" data set). To cut to the chase, they use estimates of the average marginal tax rates paid by taxpayers rather than the top marginal rate that I used in the bar chart referenced above. Their overall marginal rate is made up of not just federal tax rates, but also social security tax rates, and even estimates of the state tax rates. It should be noted the Barro is an average rate, and since the average includes non-filers (who pay zero), the Barro rate is often well below the top marginal rate. The top Barro rate is 41.8% which occurred in 1981 (compared to top marginal rates of 90%+ from 1951 and through 1963). The Barro rate is also not correlated with the top marginal income tax rate (correlation going back to 1929 is -30%).


A lot of work clearly went into producing this overall marginal rate (I'm going to call it the "Barro tax rate" for simplicity). But does it explain economic growth rates any better than the top marginal rate?

I ran a quick and dirty regression...


Growth in real GDP from t to t+1 = f(Barro Tax Rate, Barro Tax Rate Squared, Top Marginal Income Tax Rate, Top Marginal Income Tax Rate Squared)


Data ran back to 1929, the first year for which real GDP was computed by the BEA. Top marginal rates came from the IRS Statistics of Income Table 23. And the Barro Tax Rate came from Table 1 of the Barro & Redlick paper. Since Barro rates are computed only through 2005, that's when the analysis stops.

Results were as follows:



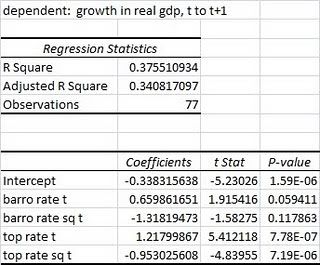
Note... the errors got big during leading up to WW2, but I don't think that invalidates this quick and dirty look.)

Here's what I get out of this:

1. There is definitely a quadratic relationship between tax rates in one period and real economic growth the next.
2. If you're going to pick either the Barro rate or the top marginal income tax rate, go with the latter. Its clearly better at explaining economic growth rates.
3. There may be something to be said about using the Barro rate and the top marginal income tax rate together. They do explain different things.

If you compute the "optimal tax rate" - the rate that maximizes economic growth implied by the regression - you get a Barro rate of 25% and a top marginal income tax rate of 64%. The optimal Barro rate was last seen in 1966, when the top marginal rate was 70% and the bottom rate was 14%. I'm guessing from this, and from looking at the Barro rate series, that this would imply that if you want to maximize growth, the top rate should be raised to about 64% and the tax burden on folks at the lower end of the income scale should be lowered. I'm not sure Barro would be pleased with these results.

I may return to this, but my next post should be the next in the series on GDP growth and the S&P 500.

As always, if you want my spreadsheet, drop me a line with the name of this post. I'm at my first name (mike), my last name (kimel - with only one m) at gmail.com. BTW... this spreadsheet contains a lot more wonky goodness!

Thanks to Sandi Saunders for getting me started wading through this particular pile of weeds.

Originally Posted by The Wonkette

But could't making steep spending cuts also throw the economy back into recession?

Government taxation and spending cannot add to GDP. They merely represent the government taking over that portion of the economy. GDP is only effected to the extent that government intervention slows down growth.

That is, unless the money the government is spending is borrowed. In which case it does add to GDP. But at what price...